Electronics Laws Overview Theories and Practical Use Cases
2024-04-12 | By Mario De Lorenzo
Introduction
Electronics is a fascinating and integral part of our modern world, powering everything from smartphones to spacecraft. Understanding the fundamental laws that govern electronic circuits is essential for both beginners and seasoned engineers. In this blog post, we'll provide an overview of some key electronics laws, including Ohm's Law, Faraday's Law, Lenz's Law, and Kirchhoff's Laws. We'll explore the theories behind these laws and delve into practical use cases to help you grasp their significance.
Ohm's Law
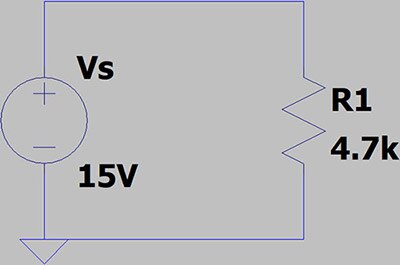
Ohm's Law, formulated by Georg Simon Ohm in the early 19th century, is one of the foundational principles in electronics. It states that the current (I) flowing through a conductor between two points is directly proportional to the voltage (V) across those two points, and inversely proportional to the resistance (R) of the conductor. Mathematically, Ohm's Law can be expressed as:
V = I ⋅ R
This law provides a cornerstone for understanding and analyzing electrical circuits.
Voltage (V): Voltage, measured in volts (V), represents the electrical potential difference between two points in a circuit. It is the driving force that propels electric charge through a conductor.
Current (I): Current, measured in amperes (A), is the flow of electric charge through a conductor. Ohm's Law states that the current is directly proportional to the voltage, meaning an increase in voltage results in a proportional increase in current, assuming resistance remains constant.
Resistance (R): Resistance, measured in ohms (Ω), is the opposition that a material offers to the flow of electric current. As per Ohm's Law, if the voltage across a resistor is constant, increasing the resistance will decrease the current flow, and vice versa.
- Practical Use Cases:
- Circuit Design: Ohm's Law is indispensable for designing circuits, determining the appropriate resistance values for components, and ensuring that the components operate within their specified limits.
- Power Dissipation: The law is crucial for calculating power dissipation (P = VI) in components, preventing overheating, and ensuring the reliability of electronic devices.
- Troubleshooting: When faced with circuit malfunctions, applying Ohm's Law helps identify issues such as open circuits, short circuits, or incorrect component values.
- Advanced Use:
- High-Frequency Circuit Design: Guiding impedance matching in radio frequency (RF) and microwave circuits to optimize signal transfer.
- Amplifier Design: Enabling the precise design of amplifiers with specific gain and input/output impedance requirements for sophisticated electronic systems.
A solid understanding of Ohm's Law is essential for engineers and enthusiasts alike, serving as a fundamental tool for analyzing and designing a wide range of electronic circuits.
Faraday's Law of Electromagnetic Induction
Faraday's Law of Electromagnetic Induction, discovered by Michael Faraday in the early 19th century, is a fundamental principle that explains how a changing magnetic field induces an electromotive force (EMF) in a closed loop. The equation EMF=−dΦ/dt quantifies this relationship, where EMF is the induced voltage and dΦ/dt represents the rate of change of magnetic flux.
Electromotive Force (EMF): EMF, measured in volts (V), represents the induced voltage in a conductor or coil resulting from a changing magnetic field. It is crucial to note that EMF is not a force but a potential difference capable of driving an electric current.
Magnetic Flux (ΦΦ): Magnetic flux is the measure of magnetic field strength passing through a surface. It is directly proportional to the number of magnetic field lines passing through the surface. The negative sign in the equation indicates that the induced EMF opposes the change in magnetic flux, adhering to Lenz's Law.
- Practical Use Cases:
- Electric Power Generation: Faraday's Law is the foundational principle behind the operation of electrical generators in power plants. Rotating turbines or other mechanical means induce a changing magnetic field, generating electrical power through the motion of a conductor within a magnetic field.
- Transformers: In transformers, Faraday's Law is employed to transfer electrical energy between coils by inducing a changing magnetic field. This allows for efficient voltage transformation in power distribution systems.
- Induction Cooking: In induction cooktops, Faraday's Law is applied to generate heat directly in the cookware by inducing a current through it, eliminating the need for traditional heating elements.
- Advanced Use:
- Induction Heating: Applied in industrial processes for metal hardening, cooking, and other applications where precise and controlled heating is required.
- Magnetic Levitation (Maglev) Systems: Utilized in transportation systems that use magnetic fields to levitate and propel vehicles, reducing friction and increasing efficiency.
Faraday's Law is a cornerstone in the field of electromagnetism, providing the basis for numerous technological advancements and innovations that shape our modern world. A profound understanding of this law is essential for engineers and researchers pushing the boundaries of electromagnetic applications.
Lenz's Law
Lenz's Law, named after the German physicist Heinrich Lenz, is a critical principle derived from Faraday's Law of Electromagnetic Induction. It articulates that the direction of the induced electromotive force (EMF) in a circuit will always oppose the change in magnetic flux that produced it. In essence, Lenz's Law ensures that the induced current creates a magnetic field opposing the original change, adhering to the conservation of energy.
When a magnetic field around a conductor changes, inducing a current, Lenz's Law dictates that the induced current will generate its magnetic field. This self-induced magnetic field opposes the original change in the external magnetic field. The negative sign in Faraday's Law accounts for this opposition, emphasizing the law's role in maintaining the conservation of energy.
- Practical Use Cases:
- Inductive Components: Lenz's Law is a crucial consideration in the design of inductive components like coils and transformers. It ensures that energy losses due to the induced current's magnetic field are minimized.
- Eddy Current Braking: In applications like eddy current braking systems, Lenz's Law is employed to generate opposing magnetic fields, slowing down and eventually stopping moving objects (e.g., trains) efficiently.
- Advanced Use:
- Magnetic Levitation (Maglev) Systems: Lenz's Law is harnessed to create a dynamic magnetic field that counters the motion of the levitating vehicle, maintaining stability.
- High-Speed Rail Systems: Employed in electromagnetic launch systems for high-speed rail, where induced currents create magnetic fields opposing the motion, providing a propelling force.
- Key Takeaways:
- Conservation of Energy: Lenz's Law is integral to ensuring that energy transformations in electromagnetic systems adhere to the principle of conservation.
- Opposition to Change: The law underscores the inherent tendency of induced currents to resist changes in the magnetic environment, influencing the behavior of inductive components in circuits.
Lenz's Law is a foundational concept in electromagnetism, contributing to the efficient design of various technologies and systems. Engineers and researchers leverage this law to optimize the performance of inductive components and develop innovative applications in transportation and energy conversion.
Kirchhoff's Laws
Gustav Kirchhoff's circuit laws are fundamental tools for analyzing complex electrical circuits. These laws are often used in combination:
Kirchhoff's Current Law (KCL)
Kirchhoff's Current Law (KCL) is a fundamental principle in circuit theory that governs the conservation of electric charge within a circuit. It states that the total current entering a junction or node in a circuit is equal to the total current leaving the junction. In other words, the sum of currents at any point in a circuit is zero, reflecting the principle of charge conservation.
∑ Iin = ∑ Iout
- Practical Use Cases:
- Current Distribution: KCL is essential for analyzing and predicting how current distributes among parallel branches in a circuit.
- Node Analysis: It simplifies the analysis of complex circuits by providing a concise mathematical expression of current relationships at nodes.
- Advanced Use:
- Integrated Circuit Design: Ensuring precise current distribution among various components in complex semiconductor devices.
- Network Analysis: Facilitating the understanding of intricate electrical networks in telecommunications and power systems.
Kirchhoff's Voltage Law (KVL)
Kirchhoff's Voltage Law (KVL) is another cornerstone principle in circuit theory. It states that the total voltage around any closed loop in a circuit is equal to the sum of the voltage drops across the components in that loop. In simpler terms, the total energy supplied in a closed loop is equal to the total energy consumed.
∑ Vloop = ∑ Vdrops
- Practical Use Cases:
- Voltage Distribution: KVL aids in understanding how voltage is distributed across various components in series.
- Loop Analysis: It simplifies the analysis of complex circuits with multiple components connected in series.
- Advanced Use:
- Signal Processing: Analyzing the voltage distribution in the loops of complex signal processing circuits.
- Control Systems: Understanding and designing feedback loops in control systems by considering voltage relationships.
- Key Takeaways:
- Conservation Laws: Both KCL and KVL are based on the conservation of charge and energy, respectively.
- Solving Complex Circuits: These laws provide powerful tools for analyzing and solving complex electrical circuits, enabling engineers to predict and control circuit behavior accurately.
Understanding Kirchhoff's Laws is crucial for engineers, as these laws provide a systematic and comprehensive approach to circuit analysis. Whether in basic circuit design or advanced applications, KCL and KVL are indispensable for navigating the complexities of electrical networks with precision and efficiency.
Tips for Electronics Projects:
- Document Your Work:
- Maintain a detailed project log, noting schematics, component values, and observations. This documentation helps with troubleshooting and replication.
- Documenting Your Electronics Projects
- Use Simulation Software:
- Utilize circuit simulation software like SPICE to test your designs virtually before building physical prototypes. This can save time and resources.
- Arduino Simulators for Hobbyists, Makers, and Classroom Environments
- Safety First:
- Always follow safety precautions, especially when dealing with high voltages or hazardous materials. Use appropriate protective gear and safety measures.
- Component Selection:
- Choose components carefully, considering factors like temperature range, tolerances, and longevity, especially in projects intended for industrial or critical applications.
- Rectifier Component Selection
- Buck Converter Circuit Component Selection
- Improving Efficiency in DC-DC Converters Through Careful Component Selection
- How to Select a Power Supply for Your Project
- There Are No Ideal Inductors
- Collaborate and Seek Feedback:
- Share your projects with online forums, local maker spaces, or online communities. Feedback from others can lead to improvements and creative solutions.
- Maker.io
- TechForum
- Explore Advanced Materials:
- Experiment with advanced materials like superconductors, nanomaterials, or specialized semiconductors for cutting-edge projects.
- Learn Advanced Techniques:
- Study advanced techniques in areas like microelectronics, nanotechnology, or quantum computing to expand your project capabilities.
- Stay Updated:
- Continuously update your knowledge by reading research papers, attending workshops, and following emerging trends in electronics.
- Applications & Technologies
- Articles
- Product Training Modules
- Training & Events
- Video Library
- Plan for Scalability:
- If your project has commercial potential, plan for scalability by considering factors like cost reduction, component availability, and ease of mass production.
- Startups Survival Guide
- Startup Roadmap
- Roadmap Dashboard
- Design Service Provider
- Patent Consideration
- For innovative projects, explore the possibility of patenting your inventions to protect your intellectual property.
These fundamental electronics laws are the building blocks upon which all electronic circuits are constructed. Whether you're a beginner eager to understand the basics or an experienced engineer looking to refine your knowledge, a firm grasp of Ohm's Law, Faraday's Law, Lenz's Law, and Kirchhoff's Laws is crucial for success in the world of electronics. With these laws at your disposal, you'll be better equipped to design, troubleshoot, and innovate in the ever-evolving field of electronics.
Remember that electronics is a dynamic field, and staying curious and adaptable is essential. Whether you're a beginner or an experienced engineer, the pursuit of knowledge and the willingness to explore new technologies and ideas will be the keys to success in your electronics projects.
Have questions or comments? Continue the conversation on TechForum, DigiKey's online community and technical resource.